Rectangular Prism Edges Faces Vertices

A rectangular prism is a three-dimensional solid shape with 6 faces that including rectangular bases.

A cuboid is also a rectangular prism. The cantankerous-section of a cuboid and a rectangular prism is the aforementioned.

Real-Life Examples

A pencil box and a volume are rectangular prisms. It may surprise yous, but your room is also a cuboid or a rectangular prism.

Properties of a Rectangular Prism:

A rectangular prism has eight vertices, 12 sides and six rectangular faces.
All the opposite faces of a rectangular prism are congruent.
A rectangular prism has a rectangular cross department.

Types of Rectangular Prisms

There are two types of rectangular prisms:

Right Rectangular Prism: The angle formed by the faces with any of its bases is 90° or a right angle. All the faces, including the lateral ones, are rectangular.
Non-Correct (Oblique) Rectangular Prism: The faces of such a prism are not at correct angles to the bases. The shape of each face is more like a parallelogram than a perfect rectangle.

Surface Area and Volume of a Rectangular Prism:

The rectangular prism has 3 dimensions. It means that it has a surface surface area and volume.

The rectangular prism has three dimensions

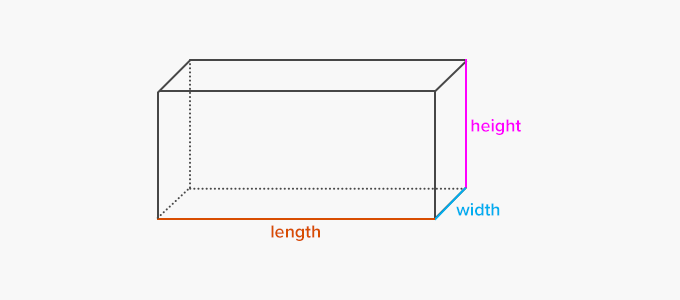
Since all the faces of a rectangular prism are rectangles and opposite faces are equal, the expanse of a rectangular prism can be calculated using the post-obit formula:

Total Surface Area $= two {(\text{width} \times \text{length} ) + (\text{length} \times \text{peak}) + (\text{width} \times \text{height})}$

Lateral Surface area $= 2 {( \text{length} \times \text{height} ) + (\text{width} \times \text{superlative} )}$

Volume of a rectangular prism is only obtained by multiplying all three dimensions $–$ length, superlative and width.

Volume $= \text{length} \times \text{width} \times \text{height}$

Fun Facts

– Rectangular prisms are the about unremarkably used prisms in existent life, specially in packaging, from cereal boxes to cartons and parcels delivered past mail.

Solved Examples

Find the volume of a right rectangular prism whose length $= 8$ cm, width $= five$ cm, and height $= 16$ cm.

Book $=$ (LWH)

$= 8516$

$= 640$ cm^three

What is the total surface surface area of a right rectangular prism with length $= 5$ feet, width $= iv$ anxiety, and summit $= half-dozen$ anxiety.

Total surface surface area $= 2 { (\text{WL})+(\text{LH})+(\text{WH})}$

Total surface area $= 2 {(45)+(56)+(46)}$

$= 2(xx+30+24)$

$= 148$ ft²

The dimensions of a rectangular prism are length = two.5 cm, width = 4.five cm, and height = ane.5 cm. Notice the volume.

Volume $=$ LWH

$= 2.5 4.5 1.five$

$= 16.875$ cm³

For a rectangular prism, length $= 4$ feet, width $= 4$ feet and height $= nine$ feet. Notice the lateral surface area.

Lateral surface surface area $= two { (\text{LH})+(\text{WH})}$

Lateral surface area $= two { (49)+(49)}$

$= ii ( 36+36 ) = 144$ ft²

Practise Problems

What is a Rectangular Prism? Definition With Examples

Attend this quiz & Test your noesis.

$16 \text{cm}^iii$

$12 \text{cm}^3$

$44 \text{cm}^three$

$10 \text{cm}^3$

Correct answer is: $16 \text{cm}^iii$
Volume $= two \times 2 \times iv = 16 \text{cm}^3$

45 cm

iii cm

5 cm

9 cm

Correct respond is: 5 cm
$h$ $= \frac{V}{(fifty \times w)} =\frac{l}{(2\times5)}=v$ cm

$248 \text{cm}^2$

$124 \text{cm}^ii$

$100 \text{cm}^2$

$50 \text{cm}^2$

Correct answer is: $248 \text{cm}^ii$
Total Surface Expanse $=$ $ii{(4\times6)+(6\times10)+(x\times4)} =248 \text{cm}^2$

$248 \text{cm}^2$

$154 \text{cm}^2$

$100 \text{cm}^2$

$50 \text{cm}^2$

Correct answer is: $154 \text{cm}^2$
Lateral Area $=$ $2\times{ (v\times7)+(half-dozen\times7) } = 154 \text{cm}^2$

Frequently Asked Questions

How many bases does a rectangular prism have?

A rectangular prism has two congruent and parallel rectangular bases.

What is the net of a rectangular prism used for?

The net of the rectangular prism is used to find the total surface area.

Can a right rectangular prism have a square face?

Yes. Some prisms are named co-ordinate to special properties. A correct rectangular prism with a square base of operations is called a right square prism.

What makes a correct rectangular prism dissimilar from an oblique rectangular prism?

The bases of the right rectangular prism are perpendicular to each other. Whereas the bases of the oblique rectangular prism are not perpendicular.